Study Guide: PI
What is PI?
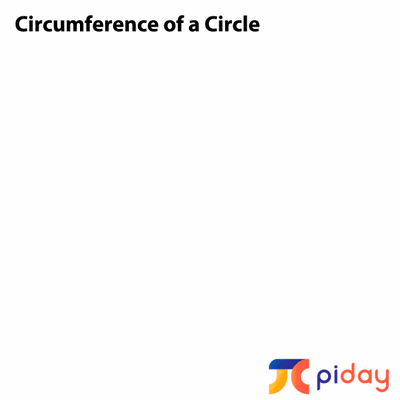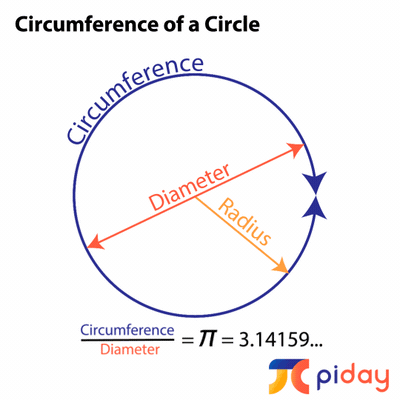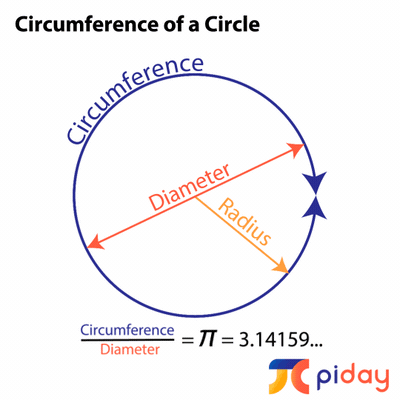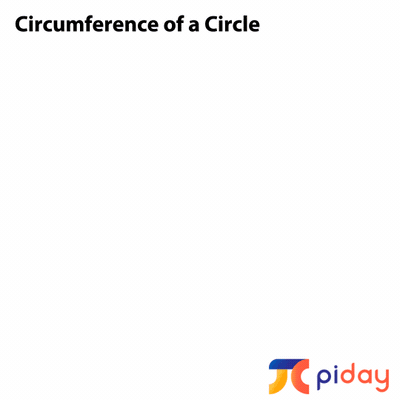
Pi (Greek letter “$\pi$”) is the symbol used in mathematics to represent a constant—the ratio of the circumference of a circle to its diameter—which is approximately $3.14159$.
$\pi$ has been calculated to over 50 trillion digits beyond its decimal point. As an irrational and transcendental number, the digits will continue infinitely without repetition or pattern. Only a few digits are needed for typical calculations, $\pi$’s infinite nature makes it a fun challenge to memorize, and to calculate as many digits as possible.
The search for PI
Pi has interested people around the world for over 4,000 years. Many mathematicians—from Fibonacci, Newton, Leibniz, and Gauss, to lesser-known mathematicians—have toiled over the mysteries off $\pi$, calculated its digits, and applied it to numerous areas of mathematics. Some spent much of their lives trying to calculate just a few more digits.
The earliest calculations of $\pi$ were based on the measurement of actual physical objects. They were discovered in many different ways. For example, in ancient Babylon, rope stretchers marking the locations of buildings and boundaries estimated $\pi$ to be $\frac{25}{8} = 3.125$. The ancient Egyptians determined the ratio to be $(\frac{16}{9})^2 ≈ 3.16$.
Archimedes
Archimedes, a Greek mathematician, is the first person known to have used an algorithmic method to calculate $\pi$. He drew a polygon inside a circle and drew a second polygon outside of the circle. Then he continuously added more and more sides of both polygons, getting closer and closer to the shape of the circle. Having reached 96-sided polygons, he proved that $\frac{223}{71} < \pi < \frac{22}{7}$. This amazingly close estimate is far more accurate than most people in his time or our’s need.
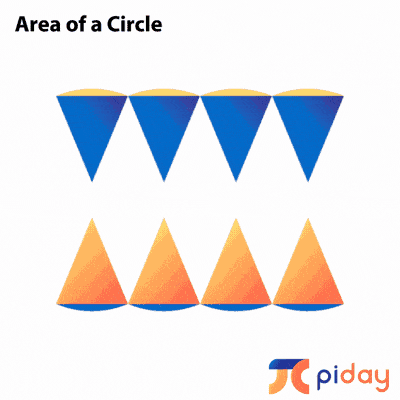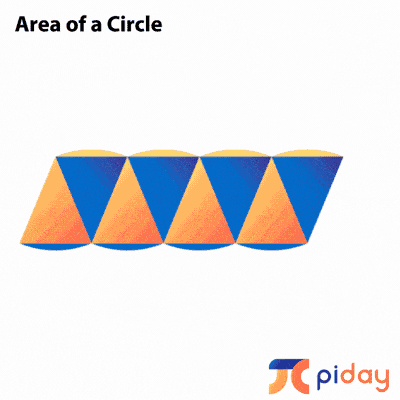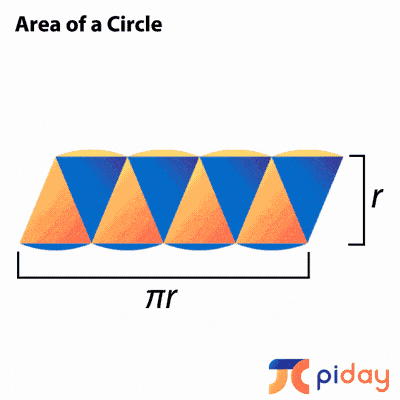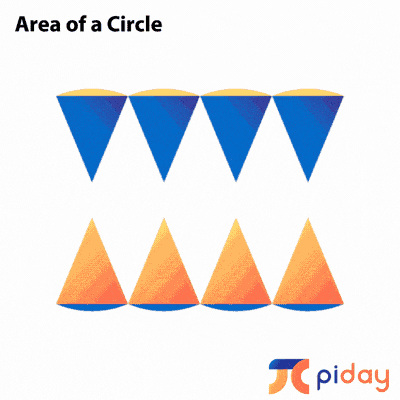
The Irrationally Transcendental PI
In 1767, Swiss mathematician Johann Heinrich Lambert proved $\pi$ isirrational and in 1882 Ferdinand von Lindemann proved $\pi$ is transcendental, which means $\pi$ cannot be a solution to a polynomial equation with rational coefficients.
Squaring the Circle
This finding is significant because, until this point, it was believed that one could construct a square and a circle with equal area, known as “squaring the circle”. Proving the transcendence of $\pi$ showed this is not possible and the phrase “squaring the circle” is now used as a metaphor for trying to do something that is impossible.
With modern technological advances, $\pi$ has been calculated to 31 trillion digits! However, only the first 39 or so are needed to be able to perform all calculations in our observable universe with virtually no error. Though it makes the news every time the $\pi$ digit record is broken, mathematicians now use technology to explore other aspects of $\pi$. One example from the Chudnovsky brothers, a pair of American mathematicians:
We are looking for the appearance of some rules that will distinguish the digits of PI from other numbers. If you see a Russian sentence that extends for a whole page, with hardly a comma, it is definitely Tolstoy. If someone gave you a million digits from somewhere in PI, could you tell it was from PI? We don't really look for patterns; we look for rules.
The Chudnovsky Brothers
PI is Everywhere?
$\pi$ occurs in many areas of mathematics. Here are a few examples from 2D and 3D geometry:
PI
$$\pi = \frac{𝐶}{𝑑}$$
Circumference of a Circle
$$C = \pi d$$ $$C = 2 \pi r$$
Area of a Circle
$$A = \pi 𝑟^2$$
Surface of a Cylinder
$$ 2\pi 𝑟^2 + h(2\pi r)$$
Volume of a Cylinder
$$ \pi 𝑟^2h $$
Surface Area of a Sphere
$$ 4\pi 𝑟^ 2$$
Volume of a Sphere
$$ \frac{4}{3} \pi ^3 $$
Surface Area of a Cone
$$ \pi rl + \pi 𝑟^2 $$
Volume of a Cone
$$ \frac{1}{3}\pi 𝑟^2h $$